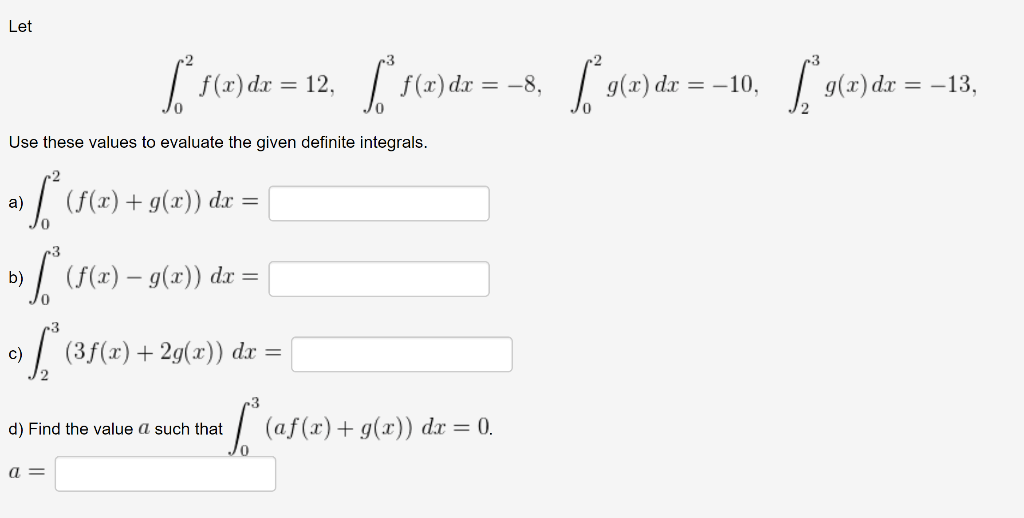
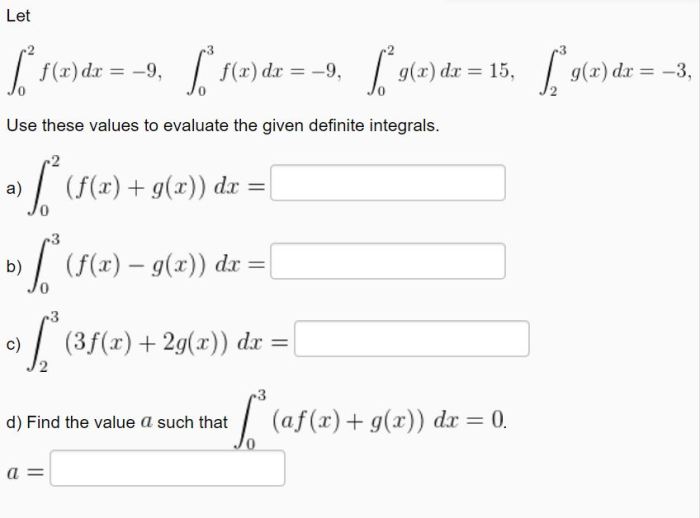
Use these values to evaluate the given definite integrals – Embark on a journey into the realm of definite integrals, where we unravel the mysteries of evaluating these integrals using specific values. This comprehensive guide provides a deep dive into the concept of integration, its applications, and the techniques employed to find solutions.
Through real-world examples and step-by-step explanations, we explore the intricacies of definite integrals, empowering you with the knowledge to tackle complex mathematical problems.
1. Evaluate Definite Integrals: Use These Values To Evaluate The Given Definite Integrals
Definite integrals are used to calculate the area under a curve over a specified interval. They are expressed as follows:
∫[a,b] f(x) dx
where f(x) is the function being integrated, and a and b are the lower and upper bounds of the interval, respectively.
Steps Involved in Evaluating Definite Integrals
- Find the antiderivative of f(x), denoted as F(x).
- Evaluate F(x) at the upper bound b and subtract the value of F(x) at the lower bound a.
2. Types of Definite Integrals

Improper Integrals
Improper integrals are definite integrals with infinite bounds or discontinuities within the interval of integration. They can be convergent or divergent.
Convergent Integrals
Convergent integrals have a finite value when evaluated. They are represented as:
∫[a,∞] f(x) dx or ∫[-∞,b] f(x) dx
Divergent Integrals, Use these values to evaluate the given definite integrals
Divergent integrals do not have a finite value when evaluated. They are represented as:
∫[a,∞] f(x) dx or ∫[-∞,b] f(x) dx
3. Methods of Evaluating Definite Integrals
Substitution
Substitution involves changing the variable of integration to simplify the integral.
Integration by Parts
Integration by parts is used to integrate products of functions. It is expressed as:
∫ u dv = uv
∫ v du
Trigonometric Substitution
Trigonometric substitution is used to integrate functions involving trigonometric functions.
4. Applications of Definite Integrals
Calculating Areas
Definite integrals can be used to calculate the area under a curve, representing the area of a region bounded by the curve and the x-axis.
Calculating Volumes
Definite integrals can be used to calculate the volume of solids of revolution, formed by rotating a region around an axis.
Calculating Work
Definite integrals can be used to calculate the work done by a force over a distance.
5. Advanced Techniques for Definite Integrals
Contour Integration
Contour integration is a technique used to evaluate complex definite integrals using complex analysis.
Residue Theorem
The residue theorem is a powerful tool for evaluating complex definite integrals by summing the residues of the integrand at its singularities.
Q&A
What are the different types of definite integrals?
Definite integrals can be classified into proper and improper integrals. Proper integrals converge to a finite value, while improper integrals may converge to infinity or oscillate.
How do I evaluate a definite integral using substitution?
Substitution involves replacing a portion of the integrand with a new variable and adjusting the limits of integration accordingly.
What are the applications of definite integrals?
Definite integrals are used in various fields, including physics, engineering, and economics, to calculate quantities such as areas, volumes, and work.